(New Instrument of Threes)
- page seven -
(The Study of Threes)
http://threesology.org
>Kurt Gödel (1906 - 1978) said the same thing from a mathematician's perspective called Gödel's Theorem which states the futility of attempting to set up a complete axiomatic formulization of mathematics [in that "truth" is evident without need of proof or argument]: In 1931 Gödel is said to have proved that any consistent mathematical system must be incomplete i.e., that in any system formulae must be constructed that can be neither proved nor disproved within that system. Moreover, no mathematical system can be proved consistent without recourse to axioms beyond that system.
Note: philosophically speaking, without even having to look at Gödel's mathematics on the subject of the futility of a "complete" axiomatic system, the answer is so stark raving simplistic it's a wonder anyone would need to develop a formula to verify such a view. It's like knowing the Sun is coming up in the morning, even if you can't see it because of a cloudy sky. I can't imagine so very many mathematicians thinking they were working within guidelines which produced its own proofs, yet the guidelines themselves were never subjected to any larger perspective of objectivity. The truth they were working with was an isolated one and not a "Unified Field" comprehensiveness.
Aside for the adjunctive puerility which symbolically retraces an adolescent's discursive entrainment involving theological questions, such as the ability or inability of verifying the proof about a God, Jesus, the Bible and other such concerns; its merit lays in the point of identifying the existence of a reality disunited from an ego using varying forms of intellectualization as a prime motivator for defending one's formulizations and placing them on a pedestal. Gödel expressed an intellectual humility that is far different from a mere emotional one. However, it is a common idea played out in varying ways which might be illustrated. But before doing so, let us review some examples of how men and women are said to think differently because their brains are "wired" peculiarly specific to their gender:
 Women tend to perform better than men on
tests of perceptual speed, in which subjects must rapidly identify
matching items such as, pairing the house on the far left with its
twin. |
 Men tend to perform better on certain spatial tasks such as
those tests that involve mentally rotating an object or manipulating it in
some fashion, such as visually turning a 3-D object. |
 Women remember whether an object, or a
series of objects, has been displaced. |
 Men are good at determining where the holes punched in a
folded piece of paper will fall when the paper is unfolded. |
 Women out perform men on some tests of
ideational fluency such as those in which subjects must list objects that
are the same color, or on tests of verbal fluency, in which participants
must list words that begin with the same letter. |
 Men are more accurate than women in target-directed motor
skills, such as guiding or intercepting projectiles. |
 Women do better on precision manual
tasks-that is, those involving fine-motor coordination such as placing the
pegs in holes on a board. |
 Men do better on disembedding tests, in which they have to
find a simple shape, once it is hidden within a more complex
figure. |
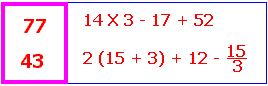 Women do better than men on mathematical
calculation tests. |
 Men tend to do better than women on tests of mathematical
reasoning.Doesn't this also suggest a type of verbal fluency? |
http://www.dhushara.com/book/socio/kimura/kimura.htm
From another perspective, here's information derived from a BBC news article:
December 2013 Last updated at 08:45 ET
Men and women's brains are connected in different ways which may explain why the sexes excel at certain tasks, say researchers.
A US team at the University of Pennsylvania scanned the brains of nearly 1,000 men, women, boys and girls and found striking differences.
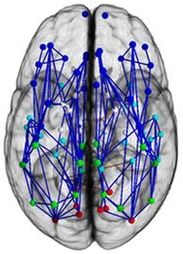
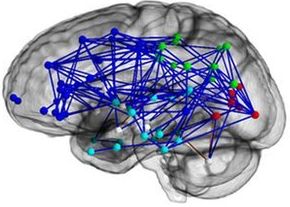
| Man's brain top and side views | |
 |
 |
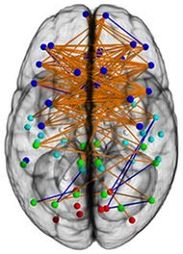
| Woman's brain top and side views | |
 |
 |
The "connectome maps" reveal the differences between the male brain (seen in blue) and the female brain (orange).
- Male brains appeared to be wired front to back, with few connections bridging the two hemispheres.
- In females, the pathways criss-crossed between left and right.
These differences might explain why men, in general, tend to be better at learning and performing a single task, like cycling or navigating, whereas women are more equipped for multitasking, say the researchers in the journal Proceedings of the National Academy of Sciences (PNAS).
And such brain differences might lead some to consider and conclude an advantaged superiority at accomplishing a given task. And the from-side-to-side brain activity might be interpreted as "scatter-brained" while the from-front-to-back brain activity might well be interpreted as tunnel-visioned, though the reader may prefer to use other labeled referencing; hopefully in a humorously spirited way. And, if given an opportunity to explore one's own gender biases based on these differentiated brain activity, the derived logic may be different as well, though either logic must nonetheless confine its "truth" to the reality of the environment where it is used. A given environment may permit the existence of different logic forms, but they are very much constricted and structured accordingly.
Whereas without living representatives of ancient peoples from different time periods, we can only speculate about the "wiring" of the brain in terms of whether it was distinctly different from those in the present era. While we know people had different ideas, we do not know if their brain was substantially different, just different in terms of knowledge type and quantity. And it can not be certain whether a language dies out just because another language comes to dominate a social setting, or if there is an accompanying change in the brain, hearing, and speaking/articulation abilities. We do know that, with respect to thinking that a person in a primitive setting does not necessarily mean their brain is substantially different from the brains of those in a modern setting. Though "wiring" connectivity may be different with respect to complexity between a simple and modern setting, this does not mean an infant from a primitive setting can not acquire the same thinking skills as those in a (presumably) more civilized setting.
A simple observation to make about patterns-of-three, although more varied and widespread in civilized settings, they none-the-less occur in primitive settings as well,; and must thus be recognized for the model in which they are presented in context. For example, the usage of a triangle spear or arrowhead are patterns-of-three. While some artistic perception might prefer to dismiss the notion of the "three" and view it as a flat-faced cone, to which the native American tepee, ant-lion burrows, termite mounds, etc., could, in their mind, be better distinguished, I won't try to belabor a point that can clearly accommodate both perceptions. Additionally, another point is to relate the continuing recurrence of the three in social forms with that which occurs biologically, namely, DNA's triplet coding, though this can well be viewed as a 3 to 1 ratio as well when the "odd man out" is included such that DNA has adenosine - cytosine - guanine plus thymine and RNA has the same first three but with uracil as the odd man out. In short, a "three" occurrence takes place on both primitive and complex structural levels of living process.
In terms of Gödel's Theorem being used, even though most are not aware of such from a mathematician's perspective, but nonetheless indicates that his idea is, for the most part, an unarticulated perception... that no doubt existed centuries ago as it does today in era-specific forms. As with expressions of the "three" differing in form and function with respect to primitive and modern contexts, they exist though expectations aligned with modern perceptions sometimes make such occurrences difficult to see, and sometimes imposes a modern "three" concept on ancient circumstances that may not have actually existed... but our organizational model makes past information easier to categorize in familiar models. But such familiarity can lead to obscurity and even arrogance when a frequency of usage becomes adopted as a standard that is used, and at times even expected to be used, as a correct procedure, and problems which may arise are not labeled as errors or wrongs, but become buried by further intellectualized elaborations. Let me provide three examples of Gödel's Theorem being expressed in contemporary events:
1. A man is asked by his wife to assemble a piece of furniture she has been asking him to put together for over a month. He decides to do it "his way", without need for referencing the instruction sheet, which is a common theme played out in many households (or garages, patios, etc.), by many men. The husband (like so many other guys) can "see" the finished project because the container not only displays what the finished product is supposed to look like, but because it is similar to other examples seen time and again in different settings. While some men eventually assemble the product correctly, others have left-over parts, others need the women's help in organizing the parts and laying out the items in a (her) logical order, and some call for help from another (male) friend who is more adept at constructing such things... of course other variations to these scenarios exist. In short, many men, and women, think they "know" the truth, the right way, and approach the problem from this axiom. And some never admit they were wrong.
2. In this second example, a women is intent on baking a cake. She opens a cookbook and refreshes her memory of what to buy and how to put the ingredients together in a certain way, including at what temperature to set the oven and for how long to bake the ingredients. After she bakes the cake and puts icing on it, she serves it to guests who come over in the evening. Everyone tells her the cake is fantastic, but she doesn't understand why she finds pieces of served cake thrown away, and no one asked for a second helping. She is disappointed and makes the remark to her husband that there was something wrong with the cake. He said that the cake was fine and would have been the "answer" for a correct dessert to be served, but the guests had all been served cake at their respective hotels. So, he amusedly said that she could have her own cake and eat it too. It was quite clear that it wasn't the authoritative text from which she derived the correct cake formula, nor how it was "constructed" or presented in a given context to an otherwise appreciative audience, but that the overall formula was wrong because it did not and could not account for all the variables. The cake was right for a given set of variables, but the rules-of-thumb (axioms) being used were the dots in a dot-to-dot game which resulted in a picture that was out-of-sync with the scenery.
3. In this third example, a young teenager takes apart an automotive engine for one reason or another, and then reassembles it based solely on his memory of disassembly only to find there are a few pieces which weren't replaced because they weren't a part of the "working picture puzzle" in his mind. He consults another authoritative (shade tree) mechanic who calls the pieces "extra" and unneeded parts put in by the manufacturer simply to increase the cost of the vehicle and to make working on the vehicle more difficult and complicated in an attempt to forcibly persuade the average person to take their vehicle into a mechanic who will charge them an arm and a leg for some simple repair. While the young man is gratified that his "authoritative" mechanically-minded friend did not deride nor belittle him for having left over parts which might otherwise signify stupidity, the young man is nonetheless concerned that the extra parts may be vital to a proper running engine; so now he takes the time to consult automotive manuals by looking up the purpose of the left-over parts by way of researching in the automotive repair reference section at a nearby public library. He concludes that the parts left off will not affect the performance of the engine as stated by his mechanic friend, and will in fact lower the weight-to-power ratio so he will be able to get more speed out of the vehicle, which corresponds with his "Hot Rod Mindedness".
4. Yet, all his efforts result in no measurable difference in speed. Thus, he begins the trek of changing "stock" parts for "performance" parts, only to find the claimed performance gains touted by different manufacturers of these after-market performance parts, yield only marginal gains in performance; as measured by a seat-of-the-pants feeling as he presses the accelerator, or because he can get from point A to point B a little faster. Whereas his "formula" for "souping up" his vehicle seems logical, he is not manifestly acknowledging how often he has to readjust his equation to include other variables... at far greater costs, financial, effort and time. Each step of the way, he must think outside the parameters of a previous formula... consulting more and more manuals, more and more advice, and establishing a "working model metaphysic" as part of an adaptive hypothesis to be tested in the field. While some may call this experience, it is a formula quite different from the one initially begun with... even though the young man (thought he) "knew" the truth of what needed to be done. In over-hearing the conversations of others beginning a similar trek as he had begun, he hears the same axioms he thought were correct, but were wrong, and there was no way to convince anyone of their false assumptions.
While most of us do not acknowledge a measurable experience of Gödel's Theorem being played out in our lives, and the level of distinction is made wholly more acute between his articulation from a mathematician's perspective and anything I might want to interject as a responsive reverberation; the fact remains that his theorem is not meant to be, or at least should not be, a singularly placed specificity to the realm of mathematics. While some readers may have a difficult time in deducing parallel applicability to other subject areas, despite any avowed numerically aligned deductiveness; there is considerable validity to Einstein's Relativity theorems with respect to perspective. Just as applied art can be, in one instance, called architecture, which necessarily involves mathematics; mathematics is not typically considered an art form in and of itself. For some, calling mathematics another art form is like calling God a clown.
And by the same token, conversationally speaking, art is not considered a form of logic nor mathematics, nor science, even when the artist stresses an intent for using these tools as foundations for their explorations in a conventionally denoted artistic expression. However, in the realm of medicine, there are some physician's who appreciate that there is both an art and science to their practice, while, in one instance, I was forced to submit a comment to a local newspaper about a practicing physician during a time I was involved with a workers compensation court case. The physician on the side of the company against my claim was making horrendous accusations based on spurious interpretations altogether having nothing to do with the injury as described, in order to use his judgment as the final word about my injury. Fortunately, I had two other opinions which agreed with my own diagnosis. The logical decisions for the (male) appeals judge was for the court to side in my behavior due to a preponderance of evidence, though the first judge decided the case against me. For all her presumed wisdom, intelligence, education and experience, she was wrong.
|
Just because a person takes the Hippocratic oath and don's a white jacket doesn't mean they are automatically endowed with a god-like superior capacity of intuition, empathy, and a bedside manner that is provocatively astute when it comes to an appropriate level of being able to accurately appreciate every single person in every single instance. Like-minded medical colleagues are not only capable of making the same correct diagnosis, but the identical incorrect assumptions as well. A medical practice is exactly what it says it is. A practice that involves the very many foibles of the human condition that medical professionals themselves are not immune from even though they work in sanitized conditions, wash their hands frequently, and wear plastic gloves. Nor should we forget that an expert opinion is sometimes referred to as educated guesstimation. No one should have to compromise their integrity by having to agree with the pronouncements of a physician when personal experience says otherwise. |
How could anyone be so smart and yet so stupid as to overlook a preponderance of evidence in a routine set of used equations applied to a multiplicity of similar problems? In the case of overlooking a preponderance of evidence for the existence of a "threes phenomena", it can be understood because of ignorance with respect to a knowledge that is not widely known and is not part of any "axiomatically" (reflexively used axiom)- derived cultural perception. So, was the judge deliberately overlooking the evidence to support a lawyer with whom she may have been friend's with and hoping I would not appeal her decision, since most lengthy law processes frequently incur even longer delays which fatigue most people because they are expecting immediate results? In any event, the analogy with a personal experience provides a "real world" example of a problem experienced by any who attempt to find a "social voice" for a personal effort such as in the case of Threes Research.
Updated Posting: Tuesday, April 22, 2014
HTML (4.01) update: Monday, June 10, 2019... 4:52 AM
Your Questions, Comments or Additional Information are welcomed:
Herb O. Buckland
herbobuckland@hotmail.com